Finding A Solution To A Recurrence Relation

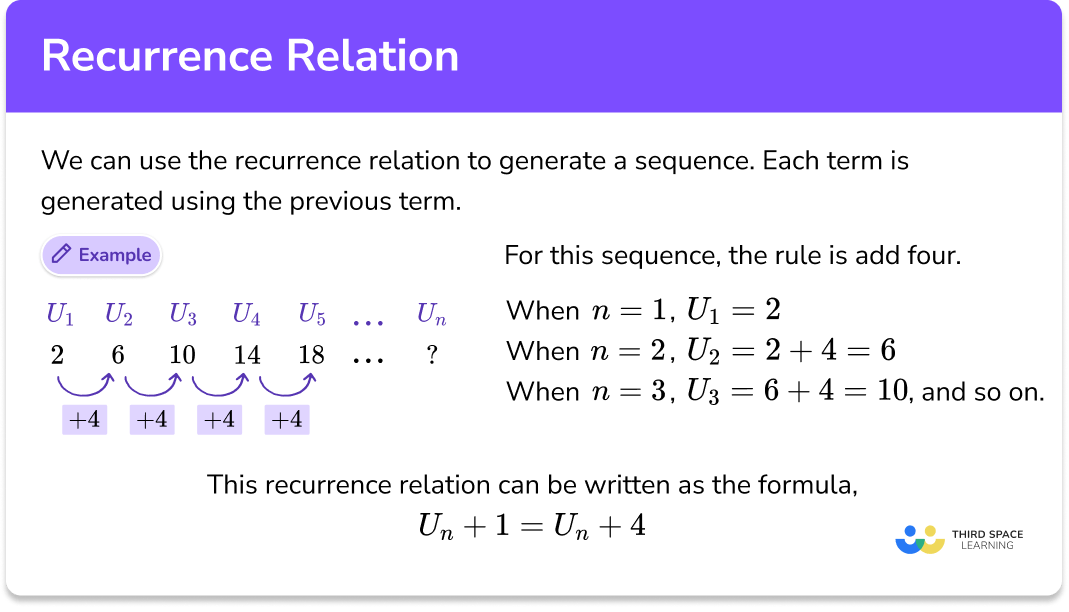
Recurrence Relation Gcse Maths Steps And Examples Solution. perhaps the most famous recurrence relation is fn = fn − 1 fn − 2, which together with the initial conditions f0 = 0 and f1 = 1 defines the fibonacci sequence. but notice that this is precisely the type of recurrence relation on which we can use the characteristic root technique. The analysis of the complexity of a recurrence relation involves finding the asymptotic upper bound on the running time of a recursive algorithm. this is usually done by finding a closed form expression for the number of operations performed by the algorithm as a function of the input size, and then determining the order of growth of the expression.

Finding A Solution To A Recurrence Relation Youtube Solve the recurrence relation an = an−1 n a n = a n − 1 n with initial term a0 = 4. a 0 = 4. solution. the above example shows a way to solve recurrence relations of the form an =an−1 f(n) a n = a n − 1 f (n) where ∑n k=1f(k) ∑ k = 1 n f (k) has a known closed formula. if you rewrite the recurrence relation as an−an−1 = f(n. Equation (8.3.1) is called the characteristic equation of the recurrence relation. the fact is that our original recurrence relation is true for any sequence of the form s(k) = b13k b24k, where b1 and b2 are real numbers. this set of sequences is called the general solution of the recurrence relation. Example: find the solution to the recurrence relation an = an 1 an 2 with initial condi tions a0 = 2 and a1 = 7. solution: the characteristic equation is r2 r 2 = 0, i.e. (r 2)(r 1) = 0. the roots are 2 and 1. thus the solution to the recurrence relation is of the form 12n 2( 1)n. since this must satisfy the initial conditions, we get: a0. 1. guess the form of the solution. 2. use mathematical induction to nd the constants and show that the solution works. 1.1.1 example recurrence: t(1) = 1 and t(n) = 2t(bn=2c) nfor n>1. we guess that the solution is t(n) = o(nlogn). so we must prove that t(n) cnlognfor some constant c. (we will get to n.

Solution Of Recurrence Relation Using Generating Function Youtube Example: find the solution to the recurrence relation an = an 1 an 2 with initial condi tions a0 = 2 and a1 = 7. solution: the characteristic equation is r2 r 2 = 0, i.e. (r 2)(r 1) = 0. the roots are 2 and 1. thus the solution to the recurrence relation is of the form 12n 2( 1)n. since this must satisfy the initial conditions, we get: a0. 1. guess the form of the solution. 2. use mathematical induction to nd the constants and show that the solution works. 1.1.1 example recurrence: t(1) = 1 and t(n) = 2t(bn=2c) nfor n>1. we guess that the solution is t(n) = o(nlogn). so we must prove that t(n) cnlognfor some constant c. (we will get to n. A recurrence relation is an equation that uses recursion to relate terms in a sequence or elements in an array. it is a way to define a sequence or array in terms of itself. recurrence relations have applications in many areas of mathematics: number theory the fibonacci sequence combinatorics distribution of objects into bins calculus euler's method and many more. recurrence. The master method gives us a quick way to find solutions to recurrence relations of the form t(n) = at(n b) h(n), where a and b are constants, a ≥ 1 and b > 1. conceptually, a represents how many recursive calls are made, b represents the factor by which the work is reduced in each recursive call, and h(n) represents how much work is done.

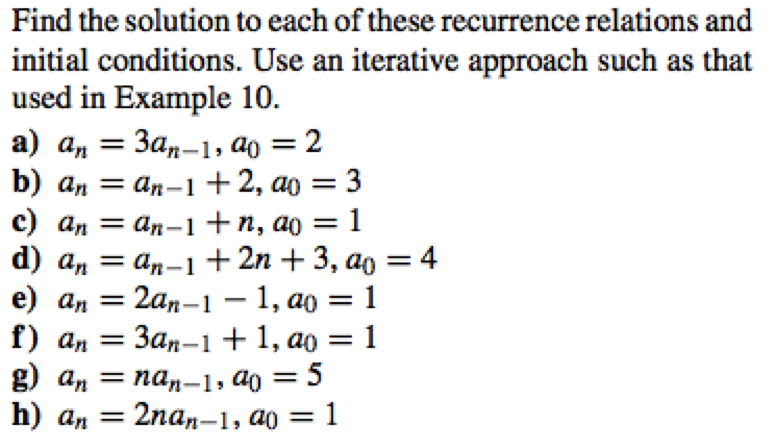
Solved Find The Solution To Each Of These Recurrence Chegg A recurrence relation is an equation that uses recursion to relate terms in a sequence or elements in an array. it is a way to define a sequence or array in terms of itself. recurrence relations have applications in many areas of mathematics: number theory the fibonacci sequence combinatorics distribution of objects into bins calculus euler's method and many more. recurrence. The master method gives us a quick way to find solutions to recurrence relations of the form t(n) = at(n b) h(n), where a and b are constants, a ≥ 1 and b > 1. conceptually, a represents how many recursive calls are made, b represents the factor by which the work is reduced in each recursive call, and h(n) represents how much work is done.