Particular Solution Of Non Homogeneous Differential Equations Mr Finding particular integral for 2nd order non homogeneous differential equations. general solution: complementary function & particular integral. using undetermined coefficients method for the particular integral. special cases & modifications to avoid overlap, obtaining particular solution. scheme of work link methods in differential equations. The complementary equation is y″ y = 0, which has the general solution c1cosx c2sinx. so, the general solution to the nonhomogeneous equation is. y(x) = c1cosx c2sinx x. to verify that this is a solution, substitute it into the differential equation. we have. y′ (x) = − c1sinx c2cosx 1.
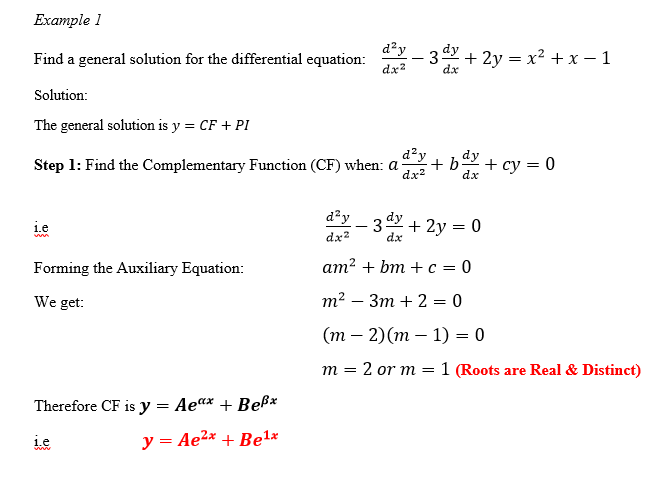
Non Homogeneous Second Order Differential Equations Math Minds Academy Example 2. find the general solution of the non homogeneous differential equation, y ′ ′ ′ 6 y ′ ′ 12 y ′ 8 y = 4 x. solution. our right hand side this time is g ( x) = 4 x, so we can use the first method: undetermined coefficients. It’s now time to start thinking about how to solve nonhomogeneous differential equations. a second order, linear nonhomogeneous differential equation is. y′′ p(t)y′ q(t)y = g(t) (1) (1) y ″ p ( t) y ′ q ( t) y = g ( t) where g(t) g ( t) is a non zero function. note that we didn’t go with constant coefficients here because. We’ll now consider the nonhomogeneous linear second order equation. y ″ p(x)y ′ q(x)y = f(x), where the forcing function f isn’t identically zero. the next theorem, an extension of theorem 5.1.1, gives sufficient conditions for existence and uniqueness of solutions of initial value problems for equation 4.5.1. Undetermined coefficients. the trick is to somehow, in a smart way, guess one particular solution to \(\eqref{2.5.1}\). note that \( 2x 1 \) is a polynomial, and the left hand side of the equation will be a polynomial if we let \(y\) be a polynomial of the same degree.
A Find A Particular Solution To The Nonhomogeneous Differential We’ll now consider the nonhomogeneous linear second order equation. y ″ p(x)y ′ q(x)y = f(x), where the forcing function f isn’t identically zero. the next theorem, an extension of theorem 5.1.1, gives sufficient conditions for existence and uniqueness of solutions of initial value problems for equation 4.5.1. Undetermined coefficients. the trick is to somehow, in a smart way, guess one particular solution to \(\eqref{2.5.1}\). note that \( 2x 1 \) is a polynomial, and the left hand side of the equation will be a polynomial if we let \(y\) be a polynomial of the same degree. Introduction. we have now learned how to solve homogeneous linear di erential equations. p (d)y = 0. when p (d) is a polynomial di erential operator. now we will try to solve nonhomogeneous equations. p (d)y = f (x): recall that the solutions to a nonhomogeneous equation are of the form. y(x) = yc(x) yp(x); where yc is the general solution to. The superposition principle makes solving a non homogeneous equation fairly simple. the final solution is the sum of the solutions to the complementary function, and the solution due to f(x), called the particular integral (pi).
Solved Find A Particular Solution To The Non Homogeneous Differential Introduction. we have now learned how to solve homogeneous linear di erential equations. p (d)y = 0. when p (d) is a polynomial di erential operator. now we will try to solve nonhomogeneous equations. p (d)y = f (x): recall that the solutions to a nonhomogeneous equation are of the form. y(x) = yc(x) yp(x); where yc is the general solution to. The superposition principle makes solving a non homogeneous equation fairly simple. the final solution is the sum of the solutions to the complementary function, and the solution due to f(x), called the particular integral (pi).
Comments are closed.